Goldmeier: The Phenomenal Content of Similarity and the Structure of Visual Objects

Goldmeier (1937) gives another example of how phenomenology is embedded in the experimental research into perceptual experience. Goldmeier claims that similarity (Ahnlichkeit) is a fundamental category of perception and conceptual cognition. In ordinary experience, similarity is actually perceived and its visual effects are compelling. Since the perceived similarity founds the ordering and sets the boundaries of phenomena, it becomes a principle of classification. Goldmeier remarks, however, that the objective (sachlich) grounds of the perceived similarity are not accessible to perceivers (1937: 147). Besides, it is not satisfactory to reduce the concept of similarity to the fact that any two objects share a feature, because any object has a feature in common with any other without being similar to it. His research then tries to specify the phenomenal content of similarity.

The contribution of phenomenology comes out for theoretical and experi­mental questions (1937: 149). Since naive subjects are able to see similarity, they are asked to report the degree of similarity of two figures in comparison with a target figure of which they are a modification. The figures appear ordered along the direction in which their perceived similarity decreases. Goldmeier concedes that this method requires that the magnitude of perceived similarity admits all possible degrees that should be ordered in a series according to the intensity, while it seems to not actually be the case. Unlike the temperature scale or the forms of order of numbers, there is no arbitrarily minimal degree of similarity that subjects can recognize; rather one may suddenly perceive dis­similarity in a smooth decrement of similarity. However, he emphasizes that this happens in every ordering in series that involves qualitative features, like coupling the order of colors with the scale of the wavelengths as well as rep­resenting quantities through the qualitative ordering of numeric magnitudes (Goldmeier refers to Wertheimer (1912b) on the perception of quantities). At any rate, this method allows defining a rank order just as if perceived similar­ity admitted of a homogeneous and continuous scale. It also provides a means to test the competing theories on the phenomenal content of similarity. The series of figures are constructed so that subjects see the change in the amount of similarity as well as its direction. A little change of the feature, which is indi­viduated by a theory as the content of similarity, is brought about in one of the comparison figures, while the other figure undergoes a greater change of the same kind. If the subjects see that the similarity between the first figure and the test figure has decreased so that the direction of similarity is reversed now towards the second figure, then the theory is false.

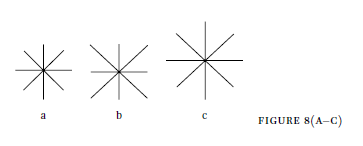
Goldmeier presents two standard theories of similarity to test whether they capture the phenomenal content of similarity in the case that their predic­tions live up to the perception of similarity of figures undergoing a particular change. The first theory predicts that the wider is the range of the parts that fig­ures have in common or the narrower are the limits of their variation, the more the degree of similarity increases (1937: 153). Let’s construct a series of figures whose perception might be either an expected instance or a counterexample of this theory, according to Goldmeier’s own experiments. Starting from the target figure 8(a), one can obtain the figure 8(b) by means of a small change in the length of its oblique lines, and then the figure 8(c) by a greater amount of change that now regards also the length of the other two lines. This series shows that 8(c) is more similar to the target than 8(b) despite having fewer parts that vary over a greater range.

The second theory predicts that a proportional variation of all parts of a figure brings about more similar figures than a non-proportional one, and that the less the non-proportional variation departs from proportionality, the less the similarity decreases (1937: 156). In order to test the perceptual validity of this prediction, Goldmeier (1937: 164-165) constructs series of figures which must be ranked according to their degree of similarity with respect to the target figure by the subjects, who are sometimes allowed to make multiple choices. In such a multiple choice task, they select the comparison figure that is more similar to the target, then drop it out of the set of the available figures and choose another figure until the last comparison between the only two figures left has been made. Contrary to the second standard theory, Goldmeier found that the figures that underwent a proportional variation under every respect were ranked as those which appeared less similar to the target. The follow­ing series provides an example constructed according to the same conditions tested by Goldmeier.

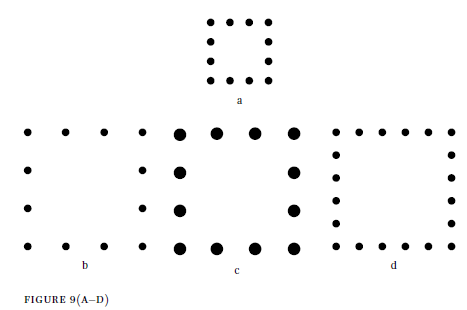
The comparison figures 9(b-d) are all twice the size of the target square 9(a). The figure 9(c) is the only one that results from a proportional doubling of the target under every respect, while the dots in 9(b) have the same size as the dots in 9(d), whose number moreover is greater than that of the dots in 9(a). Yet 9(c) does not appear as more similar to the target.

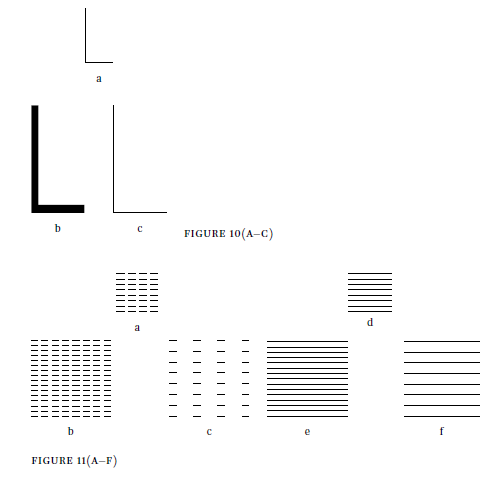
Goldmeier claims that this evidence suggests taking into account the phe­nomenal features of figures that may regard the material and the form of vi­sual objects (1937: 166, 174, 180). The particular size and distance of elements are examples of material features. The structure of the material, for instance being arranged in a straight line or in a circle, is an example of a formal fea­ture. The squares in figure 9 show that this distinction between material and form is appropriate. It accounts for the fact that the greater number of dots of the same size allows 9(d) to preserve the density required by the straight outline of 9(a). Then material and formal features can be considered features of the perceived similarity insofar as their variation brings about a change in it. This implies that both are taken at their perceptual face value. For example, the thickness of a brush mark is not usually perceived in connection with its length with respect to the width of the sheet on which the stroke is drawn. This material feature is only a geometric property that may not have a phe­nomenal realization in the stroke. Therefore, its variation will have no influ­ence on the perceived similarity. Instead, if this geometric property is realized in the phenomenal material of the figure, a variation of thickness will bring about a decrement of similarity. This holds also for the gaps between the ele­ments in the figure 9(a). With the restriction that geometric properties may be relevant only if phenomenally realized, the proportionality theory can be rephrased thus: the similarity between figures is preserved if the variation of the phenomenal formal features is proportional and the material features are kept constant. Another example is figure 10, which shows that the distinction between phenomenal form and phenomenal material applies also to continu­ously connected figures.

This new version of the theory explains why 10(c) is seen as the most simi­lar figure to 10(a)even though its aspect ratio in comparison with 10(b) actu­ally departs from proportionality, because the thickness is not realized in 10(a) as a perceptual material feature, while the length is realized as its perceptual formal feature. Goldmeier (1937: 168) states the conditions under which the properties of visual objects, whether they are continuous or consist of discrete elements, are perceptually distinguished into formal and material features. El­ements that are (1) comparatively very small and disjointed from one another, and (2) in such great a number that they do not appear as individualized parts, become material properties, which do not bear a phenomenal relation to the composition of the whole object. The following two series of figures prove the condition (2).

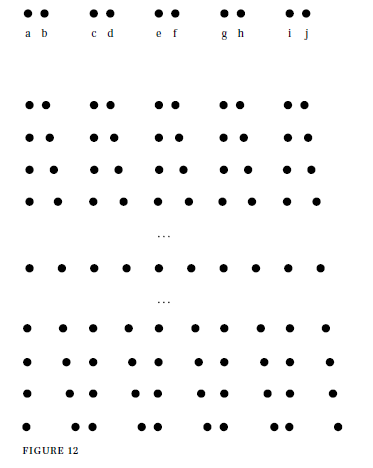
In both series u(a-c) and u(d-f), the comparison figures are obtained by doubling the linear dimensions of the target figures 11(a) and 11(d) in such a way that the number of their elements can be varied while keeping their size constant. In both cases, the more similar figure to the target is the one in which the number of elements has been increased, namely 11(b) and 11(e). The targets 11(a) and 11(d) are composed of so many elements, be they continuous or not, that their appearance as a material property reaches what Goldmeier calls an “accumulation” point, from which it becomes a structural property of the vi­sual object. For this reason, when the target figure undergoes an enlargement, the number of the elements is expected to increase accordingly to preserve the composition of the figure, even if the geometrical proportion between the target and the enlarged figure under every respect is not met. For instance, the distance between the elements is preserved only in 11(c) and 11(f). Instead, the increase in the number of elements in the figures 11(b) and 11(e) makes each element appear not as an individualized part but rather as a piece of a material that belongs to and is spread over the whole object. By presenting more elements than the figures 11(c) and 11(f), 11(b) and 11(e) are more simi­lar to the targets whose elements’ accumulation makes the property of each element lose any connection to the visual object, while their density and or­dering as a whole has become a structural feature of it.

The distinction between phenomenal material or formal features and geo­metric properties enables Goldmeier to bring in another part of the phenom­enal content of similarity, namely the structure of figures, by means of which it is possible to define the features that are most responsive to the variations that bring about a change of perceived similarity. Goldmeier (1937: 174, 192) designates as structure (1) the composition rule of a whole visual object, for example the unification of its elements according to one or various grouping factors, which specifies them as parts that have a perceptual function and into which the whole can be divided in a perceptually natural way; and (2) the par­ticular feature that realizes this rule so conspicuously that it makes the visual object an individualization or a particular realization across a range of its pos­sible perceptual specifications.

This meaning of perceptual structure derives from Wertheimer (1923), who observed that a feature comes to assume a particular value if it is perceived as the reference point of the various appearances in which it can be realized through a smooth transformation. Wertheimer (1923: 316-319) called “Pragnanzform” the composition of a visual object in which a feature appears so conspicuously that the object is perceived as a clear-cut (pragnant) realization of that sort of property. To provide an example, one can follow a suggestion of Wertheimer himself (see figure 12).

Starting from the first set of dots grouped into couples by proximity, one can obtain other rows of dots by letting the place of b between a and c or that of d between c and e, and so on, vary systematically, while holding the distances a-c, c-e, g-i constant. If the dots are as smoothly displaced as possible, for every displacement many other rows of dots appear that do not, however, have the same perceptual meaning. In an ideal matrix of the possible rows, which would include the rows in the above figure, three of them are forced upon the subjects as perceptually outstanding: a-b/c-d/… in the upper row, /b-c/d- e/… in the lower row, and finally the row that in the matrix would be equidis­tant from the upper and the lower rows. Wertheimer remarks that the rows lying in-between these three appear as if they were somehow “indeterminate,” because they do not display the grouping in the same perceptually incisive form as do these three. Therefore the upper, equidistant and lower rows ap­pear as reference points through the displacements, because they realize three perceptually clear-cut forms of composition of elements given a grouping fac­tor. All the other rows are seen instead to derive from or to approach them. The intermediate rows appear as the ranges of the possible variation of these three forms in which a composition rule is presented as clear-cut. On this basis.

Wertheimer claims that the smooth variation of the constitutive parts, or of a feature of one visual object, brings about appearances characterized by the degrees of their approximation to the clear-cut level (pragnanzstufe) in which they perceptually realize the composition rule of parts or that feature. Any clear-cut perceptual level acts as one reference point in connection to which the appearances can be ordered as more or less individualized realizations of that particular composition or feature. For example, Wertheimer reports that if the subjects are presented with angles varying between 30° and 150°, which are obtained by smoothly displacing the horizontal side of the angular sector, there will be three clear-cut levels. One will be the angle perceived as the clear- cut realization of an acute angle to which the angles seen as more or less acute are referred back, one likewise that is perceived as the clear-cut realization of an obtuse angle, and finally the appearance of the right angle. Each level has a layered organization with a central clear-cut appearance and a range of ap­proximations so that, for instance, a 93° angle appears to be an “almost” or a “bad” right angle instead of appearing as a distinctly individualized realization of an independent angular value. This means that a 93° angle belongs to the range of possible variations of the right angle and accordingly that it is per­ceived as a less individualized form of this angle.

In this connection Goldmeier (1937: 185-188, 190) points out that not every possible change of a figure will bring about a change of perceived similarity, since for a geometric property there might be a particular instance that is a clear-cut perceptual realization of it, a range of perceptual instances that ap­proximate it or even none. Then it is likely that the more a property is realized in a clear-cut perceptual feature, the more responsive is its appearance to an alteration of similarity, because this feature counts as a structural property of the figure fully specified in its appearance. The figure is not perceived as only one among a range of possible appearances of that feature. Accordingly the degree to which a figure displays the realization of (one of) its structural fea­tures is an abstractly definable part of the phenomenal content of similarity. To grasp intuitively what Goldmeier means, let’s construct some examples that meet his experimental conditions by employing the case of the perception of angles mentioned by Wertheimer.

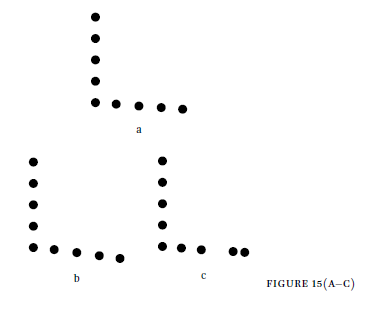
The comparison figures 13(b-c) derive from the target 13(a) by means of a displacement of the elements of the angular sector on the horizontal side. The displacement has the same amount in both figures (about 6.3 mm), but it regards the distance of the whole side from the horizontal in 13(b), while only the positions of the elements in 13(c).

In such cases, even if 13(b) thoroughly preserves the relative distance of the elements of the target 13(a), the subjects see the figure 13(c) as more similar to the latter. According to Goldmeier, 13(c) is seen as an equally clear-cut percep­tual realization of an angular value as is 13(a), while 13(b) is seen as one of the possible appearances among the range of another reference point, namely the obtuse angle. Conversely, one has to expect that diminishing the perceptual conspicuity of a feature brings about a decrease in the perceived similarity. The next figures provide a test case.

The figure 14(a) shows a clear-cut realization of a right angle as well as of an equal distance arrangement of its elements. The comparison figures are obtained, as in the previous example, by means of a displacement that amounts to the same magnitude regarding the distance from the horizontal of the whole side in 14(b) and among the elements in 14(c). For an experiment with the same conditions, Goldmeier reports that the subjects’ similarity judgements were equally divided between the two comparison figures, which appear equiva­lently similar to the target according to either feature instanced. Since both features have a full perceptual realization in the target, they are both highly responsive to the similarity perception brought to bear by a relevant change. The next figures provide the example of a final test of the contribution that the level of the clear-cut realization of a feature gives to the phenomenal content of similarity.

The target figure 15(a) is constructed to represent one of the possible ap­pearance in the range of an obtuse angle and, at the same time, to show a clear- cut realization of the feature of the equal distance arrangement that rules the composition of its elements. In a similar experimental test, Goldmeier reports that the similarity judgements of the subjects converge on figures like 15(b), although it corresponds to a more obtuse angle than 15(a) and 15(c). This dif­ference in the angular values does not affect the perceived similarity, because 15(a) and 15(b) are seen as belonging to the range of appearances of the obtuse angle, whose lesser or greater degree of approximation to this reference point is equivalent for the perception of similarity. Accordingly, this change does not affect the similarity perceptual judgement, unlike the equal distance arrange­ment, and 15(b) is seen as a similar variant of the target 15(a).

Source: Calì Carmelo (2017), Phenomenology of Perception: Theories and Experimental Evidence, Brill.