Mean Center vs. Z Score in Moderation Testing in SEM Model

Instead of creating a new variable where you are subtracting the variable’s mean, SPSS gives you another option to center the data by “standardizing” the indicators (or converting it to a Z score). With mean centering, you are changing the mean value, but the standard deviation and scale remain the same.This is not the case with Z scores. If you convert your data to a Z score, SPSS will rescale the data so that one unit in the data is equivalent to one standard deviation. Both methods will center the mean to zero, but using Z scores will also completely rescale the data.

To create a Z score with your data, you need to go to the “Analyze” menu option in SPSS, then select “Descriptive Statistics” and then choose “Descriptives”. A pop-up window will appear where you will include the variables of the moderator and the independent variable in the “Variables” option.You will also see at the bottom of the pop-up window a checkbox that states “Save Standardized Values as Variables”. Check this box and hit OK.

Figure 7.28 Creating Z Scores of Variables in SPSS

SPSS will create a new variable for each one of the variables selected in the Descriptives window. At the end of the data, SPSS will add a “Z” in the front of the newly formed variable along with the original variable name. See Figure 7.29.

Figure 7.29 Example of Variables Transformed Into Z Scores in SPSS

These standardized variables are now mean centered and rescaled.You will then use these Z score indicators to create interaction terms just like we did with the mean centered example. I have included an example of what the graphic depiction would look like in AMOS. Notice that the interaction terms are all reformed with the Z scores and all the labels have a Z at the front to denote a Z score interaction.

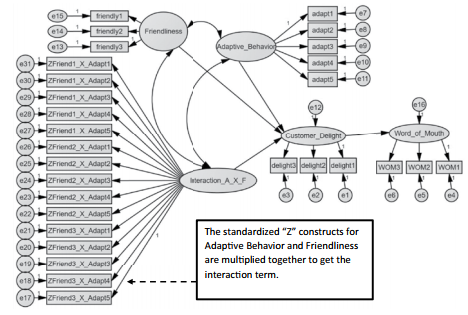
Figure 7.30 Example of Z Scores Used in Moderation Testing

Let’s now look at the results of our full structural moderation test with the standardized Z score interactions and compare the results to the mean centered moderation test.

Figure 7.31 Comparison of Mean Centered and Z Score Results

Comparison of the results shows a small difference in the unstandardized regression weights of the interaction variable.The t-value for the interaction is exactly the same across the differ- ent tests. The standardized regression weights are also exactly the same across the two tests. Any differences that are present across the two moderation tests is a result of rescaling the data with the Z scores and rounding considerations with the mean centered option.The results are essentially equivalent.

Probing the interaction with a Z score moderation test is accomplished in a slightly different manner. Transforming the data to a Z score rescales the data where 1 unit (or a value of 1) is 1 standard deviation. To create the high moderation variable, you would need to take the Z score for each indicator of the moderator and subtract a value of 1. For the low moderation, you would add a value of 1 to all the Z scores for the indica- tors of the moderator. After that, you would form your interaction terms with the Z score Hi/Low moderator indicators multiplied by the independent variables’ Z score indicators.

Whether you use mean centering or Z scores, both methods should help to account for any potential multicollinearity between the moderator and the independent variable.While the Z score option may save you some time, you need to be aware that Z scores will rescale your data and subsequently impact standard deviation values.With a mean centered value, your standard deviation does not change from the original data, and the scale is still the same. One unit with a mean centered value is still one unit.

Source: Thakkar, J.J. (2020). “Procedural Steps in Structural Equation Modelling”. In: Structural Equation Modelling. Studies in Systems, Decision and Control, vol 285. Springer, Singapore.