Meaning in the Perceptual Field: Figure-Ground and Contour in Rubin

In his work on the figure-ground structure, Rubin (1921) addresses similar questions to Brentano’s theory of boundaries and qualitative continua. Ru­bin was acquainted with the theories of the Brentano School and quotes the work of Meinong, Benussi and Husserl, together with Schumann (1900) and and Schapp (1910). This section emphasizes the extent to which Rubin’s experi­mental work on the visual contours and points, the various kinds of line- and surface-figures, may be considered a probe of the theory independently devel­oped by Brentano.

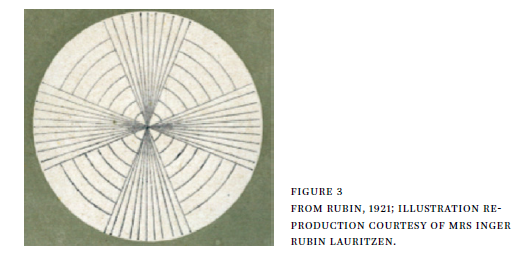
Rubin makes clear his phenomenological commitment, claiming that the figure-ground structure is not something added by the mind to the sensory data; rather, it is a perceptual given that needs a change of the visual field. Moreover this change is not due to attention that adds a sense of “distinction” (Klarheit) to sensory data, as if the appearance of a figure was the result of clos­er inspection of the data. Instead it is a phenomenal change of the function of the field that is directly experienced (1921: viii; see Koffka, 1921, who employs this argument drawn from the 1915 first Danish edition of Rubin’s work). See for example figure 3.

This pattern allows two different perceptual objects to emerge, each en­dowed with a distinct figure-ground structure. If first a figure is seen in the radially hatched cross, the concentrically round pieces do not disappear or end at the cross boundaries, rather they continue to run as inner parts of the cir­cular ground passing behind the cross. If a figure is seen in the concentrically hatched cross, then the radial straight lines have to make the inner parts of the ground, which are perceived as sectors thereof.

This makes clear that the phenomenal change underlying the figure- ground segregation and inversion is not a matter of mental integration or attention, because the same region that was previously seen as ground is re­ally visually altered and richer if seen as figure. Rubin (1921: 36-39) states that the description of such facts cannot mention the physical objects’ properties and must be based on evidence that is not simpler than that occurring in the ordinary perceptual experience. Thus it may distinguish between the percep­tual meaning of properties depending on whether they belong to figure or ground.

The phenomenal distinction between figure and ground rests on the asymme­try of boundaries that was pointed out by Brentano. Rubin (1921: 38) suggests putting a cardboard piece, previously cut to a meaningless shape, obliquely and close to a frontal-parallel uniformly colored wall. The common boundary delimits the figure only towards the cardboard surface, while the wall surface is perceived to continue behind it. The asymmetry of the common boundary underlies the distinction between figure and ground. If two areas in the visual field delimit each other and either of them is seen as figure or as ground, the asymmetry accounts for the fact that the boundary becomes the contour shap­ing a figure along one direction towards only one or the other of the two areas (1921: 36-37). The field area that touches the boundary on the side to which contour has effect becomes the figure, while the other becomes the ground. The asymmetry explains also that the contour has more meaning for the figure than for the ground so that the ground extends behind the figure. This per­ceptual meaning does not derive from prior knowledge. Rubin remarks that the task of telling which parts of the wall form the ground covered by the cardboard is difficult but not different from the task of drawing them accord­ing to perspective. Instead the task of telling how the covered parts look is “unnatural” because it is in contrast to the ordinary evidence that the common boundary cannot be seen as the contour of the ground wall. The meaning of the contour requires that the figure to which it belongs ends at it, while the ground extends behind it further across and past the contour.

Rubin (1921: 39) shows that, indeed, the perception of what happens at the boundary may be thoroughly independent of knowledge. An irregular black piece of paper is put in the middle of a white paper lying on the ground. If the surrounding white paper is seen as the figure, it is easy to see a hole in the black region that is filled by the darkness spreading behind it, as it was an aperture in the white figure. Rubin reports that to make the arms of the white figure more prominently run into the hole, he had to remove the black paper and cut it. De­spite knowing that in fact a black paper overlaid a white one, the perception of the common boundary as the closing contour of the white surface was forced so strongly on him that he struggled with the absurd idea of cutting a hole to make the surface larger. The rules that set the probability of perceiving a figure are phenomenological: the “surroundedness,” the relative size and the orien­tation (1921: 79-88). Likewise the character of the figure against the ground: the figure has a thing-like character, while the ground a stuff-like character, a nearer localization in relative depth and an enhanced brightness of its mode of color appearance (for the latter notion see Katz 1911, 1930; infra § 3.3).

Rubin discusses the asymmetry of boundary in connection with the stable appearance of the environment of ordinary experience. The higher likelihood that a common boundary appears as the contour of a surrounded region rather than the surrounding one is not only an essential feature of the perceptual forms but also of environmental things (1921: 91ff.). Their visual properties are particularly important for the acquisition of knowledge. Yet if boundaries were symmetrical, the contours couldn’t delimit the form of things, so their cogni­tion would be substantially lessened. Moreover, the asymmetry accounts for the fact that the form of things does not change as the surrounding colors do. Rubin shows that the thing-like character of the figure is connected with the color constancy which is more likely to be realized in the region appearing as figure than in the one appearing as ground (1921: 5iff.)

If the double cross in figure 4 is presented so that one white sector is lightly shaded, the shadow appears more conspicuous if the black rather than the white cross is seen. If the figure is drawn on a uniformly green ground and the subjects are given a piece of orange-red gelatine through which to see the fig­ure, the color of the figure fluctuates between a greenish and a yellowish color. If the subjects look at the cross, it stands out against the ground by means of its color, which will become a faint green that remains approximately constant against the reddish ground regardless of the sectors of the field in which the cross is seen. Without asymmetry the form would be connected on the contin­gent surrounding field, the part of it that is perceived as the ground would not continue behind the figure and the form could not convey information on the shape of environmental things. Even the belief that a thing has to be located in a delimited part of space is founded on the asymmetry. As the sight moves across the field, both the thing and the nearest adjacent neighboring region could appear as a visual form because the contour could be directed to both sides. The form would appear undetermined and the shape of things would be perceived to change at each change of place of the thing with respect to the background. Rubin concludes that without asymmetry, however regular the connection between the visual forms and the environmental things, this would be meaningless for our mental life.

Therefore, the perceptual properties of the boundaries do not depend on knowledge, rather they belong to the essential features of perception that allow one to extract the knowledge of the world in phenomenal form. Indeed, Rubin defends the independence of perception. He rejects the view that perceptual forms are mental properties that are projected unawares on external things, as well as that they are learned through association rules drawn from physi­cal regularities. The physical constitution of objects cannot account for the fact that the boundary appears as the contour of the surrounded region so that the form is perceived solely in the enclosed region. One can only assume that if perception has self-sufficient rules for forms, these must have a momen­tous value for cognition, because if a boundary appeared as the contour of the enclosing region no shape could be grasped, except perhaps ring-shaped hol­low things like vases.

According to Brentano, boundaries necessarily depend on higher dimen­sional entities and this implies that the former are perceptually distinguished from the latter. Rubin (1921: 105) emphasizes that when a common boundary appears as the contour, subjects see its effect, namely the surface standing out as a figure rather than the contour itself. Nevertheless the contour is re­ally distinguishable from the figure. When a figure appears, it becomes a sur­face with a form and defines a visual region, while the contour appears to have length but no breadth. It encloses an area that does not define a visual entity. If a field region appears alternately as figure or ground, it may appear colored in both cases, while the contour appears always colorless. Contours are also distinguished from figures by their parts. If a piece of paper is cut off to make it look like a square, its form shows no inner articulation and it is perceived as a figure without parts. Yet when subjects describe the contour of the same piece of paper, they report that it consists of four parts. Consequently, like Brentano, Rubin (1921: 135) contends that the connection between contour and figure is a mutual determination with degrees of relative independence. Every change of the whole visual thing brings about a change in the contour and the figure, but there are changes of the figure that do not alter the contour and vice versa. For instance, color changes in the figure have no effect on the contour, which has no color at all. If a figure is seen in a stripe, the changes of the edges of the stripe may have no effect for the figure, but if they alter the equal width throughout the length of the stripe, then they will have an essential influence on the figure.

In order to inspect more closely the connection between contour and figure, Rubin designs an ordered series of changes that, at one extreme, alter contour properties with little or no meaning for the figure, and at the other may af­fect the connection itself between contours and figures. The initial change of contour may consist of straightening curved lines. It may not alter the percep­tion of a figure with a round surface, as when a stamp is perceived as a square despite its jagged contour. The alteration of the figure happens if the contour is cut in plain, quite long and straight boundaries. The preservation of the form of the figure is a proof that there are contour properties that belong to it but have no role in how it is perceived. The surface of the figure appears as it were so solid and robust that it is not affected by such changes, which are perceived as minor unevenness of the contour, whether regular or not. At the opposite extreme, there are figures with surfaces that do present forms but not a distinct contour. The quite torn, thin clouds in the blue summer sky show figures with distinct surface but are so fragmented or pass so imperceptibly into the sky that they have no defined contour. The same holds for the figures occurring in the peripheral visual field, in conditions of strong adaptation to darkness and through scatter lens. Sharp contour lines can be present, but their perception still eludes us. This point is supported by the construction of the following pat­tern (1921: 138). A small white disc is put on a larger grey disc. By drawing chalk lines on the white disc and wearing suitable glasses, the white appears to pass smoothly into the grey through grey-white shades so that it is impossible to tell where the white ends and the grey begins or even to observe contrasting Mach bands. Nonetheless a round surface is still distinctly perceived. One cannot say how large it is or exactly which parts are connected with the smooth color tran­sitions. The roundedness somehow spreads across the whole area that is per­ceived as figure. Rubin reports the perception of something concentric without any clue to enable seeing the drawn circle, so that it is hard not to claim to have the visual experience of a figure with a round surface albeit with no contour.

To carry out a deep analysis of dependence, Rubin (1921: 144ff.) compares the relation holding in the whole visual thing, in which contour and figure are connected, with that between figure and ground. Figure and ground are mutually independent, because it is required only that they cannot be simulta­neously given in the same visual region. In the figure-ground inversion, either of two distinct visual objects fills one and the same region. Contours and fig­ures belong to the same visual region but do not exclude each other. They are distinguishable but connected in a whole in which they are simultaneously given as features of one and the same thing. Rubin remarks that the descrip­tion of the region in which one figure appears requires terms denoting the figure and the contour, but not the ground. Rubin (1921: 147) acknowledges that there are protocols in which the contour of meaningless figures is not men­tioned, but this may result from the different roles that the contour and the
figure play in the whole and in the relative independence of their changes. If a figure with a sharp outline is presented to a subject within a series of bluntly delimited figures, she is likely to notice this contour property. If it is presented in a series of sharply delimited figures, this property will probably be unno­ticed, although the sharpness is still a property of the contour that belongs to the figure.

Therefore, although the dependence between boundaries and higher dimensional entities remains valid, which are the roles a contour plays in the perception of the whole thing? In the figure 5 a figure is perceived either in the white or in the black field. If the subjects see the white figure as they track the curved contour and then try to see the black figure by again tracking the contour, they see that the contour belongs in the first case to the white surface and in the second to the black one. In the first case, the contour is concave, bending its hollow side to the outside. In the second, it is convex, bending its hollow side to the inside. If subjects succeed in seeing the white figure and also the convex contour, the contour becomes an independent feature of the figure and the black figure is highly likely to be seen. The contour can be either fully integrated in the whole or one of its constitutive parts. The belongingness of contours and figures actually influences how the contour is decomposed into a plurality of its own natural parts. In the pattern in figure 6 either a white or a black figure is perceived. If perceivers see the white figure and track the contour as belonging to this field region or vice versa, the contour will consist of pieces that follow one another as bounded together in pairs. However, this happens for the two pieces that enclose in the first case the white fringe and in the second case the black fringe, among which angular relations hold.

This shows that the mode in which the boundary is connected to the surface determines how the contour belongs to the figure and its parts to one another.

Rubin’s analysis deals with another key concept of Brentano’s theory, namely continuity. He considers continuity as a phenomenal feature. Indeed, a continuous line shows a set of black points with small interstices or gaps if observed through a microscope. On the other hand, if a series of black squares is presented to the subjects at a close distance, they see a line composed of discrete parts with gaps. At suitably increased distances it becomes difficult to see the single squares, and at some point these can no longer be seen. Not­withstanding the same stimulation, continuity now appears. Rubin claims that continuity is perceived if, once an arbitrary point in a visual line is selected, it is impossible to give it a particular perceptual relief or to perceive the smallest visual distance between it and the point nearest to it. Rubin’s account is con­sistent with Brentano’s claim about the coincidence of boundaries, although Rubin remarks that the established mathematical concept of continuity to some extent agrees with these observations concerning the visual lines (1921: 163). Rubin suggests that continuity is characteristic of figures consisting of lines instead of surfaces. If a black circle is drawn on a piece of paper, it is pos­sible to see a thin ring in the black drawing but also a round disc in the inner surface or a circular hole of the outer surface. If the black line is very thin, it is impossible to see it as a ground and the white paper as a figure. The thin ring is a line-figure, the round disc and the bearer of the hole are surface-figures. Of course, lines and bands also provide the foundation for surface-figures (see 1921: 161, for the two kinds of line-figures).

Rubin’s observations on the dimension of visual points and lines address the questions of Brentano’s theory of the dimensions of perceptual continua.

Rubin (1921: i6iff.) claims that any arbitrary line appears to have length, but the appearance of breadth or width is contingent upon its perceptual prop­erties and conditions of presentation. A visual line is distinguished from a visual stripe because it is one-dimensional. The one-dimensionality of a line with no width is a perceptual feature rather than an abstract property that is obtained by taking the limit of it. Suppose a line is drawn on a cardboard attached to the wall so that the line lies perpendicular on it. When it is seen from a nearby standpoint, the line has a noticeable width and the subjects can tell its right from its left side. If the standpoint is put at increasing dis­tances, a point is reached where it does not make sense to distinguish be­tween a right and a left side of the visual line. If the cardboard is attached to the wall so that the line lies horizontally on it, at increasing distances a point is reached where the distinction between an upper and a lower side makes no visual sense.

This phenomenological account allows Rubin to show that the percep­tual world admits points with no extension, thus giving indirect support to Brentano’s notions of dimensionless boundary points (see 1921: 194, for the argument that this phenomenological account is distinguished from a le­gitimate physiological one). Let a small dark surface lying on a piece of light cardboard be attached to the wall. From a nearby standpoint, it is possible to perceive the parts of this visual object that are located above, below, right and left. At increasing distances this distinction will no longer be possible, so that a visual point with no extension appears.

Source: Calì Carmelo (2017), Phenomenology of Perception: Theories and Experimental Evidence, Brill.