Dominant Strategies

How can we decide on the best strategy for playing a game? How can we deter- mine a game’s likely outcome? We need something to help us determine how the rational behavior of each player will lead to an equilibrium solution. Some strategies may be successful if competitors make certain choices but fail if they make other choices. Other strategies, however, may be successful regardless of what competitors do. We begin with the concept of a dominant strategy—one that is optimal no matter what an opponent does.

The following example illustrates this in a duopoly setting. Suppose Firms A and B sell competing products and are deciding whether to undertake adver- tising campaigns. Each firm will be affected by its competitor ’s decision. The possible outcomes of the game are illustrated by the payoff matrix in Table 13.1. (Recall that the payoff matrix summarizes the possible outcomes of the game; the first number in each cell is the payoff to A and the second is the payoff to B.) Observe that if both firms advertise, Firm A will earn a profit of 10 and Firm B a profit of 5. If Firm A advertises and Firm B does not, Firm A will earn 15 and Firm B zero. The table also shows the outcomes for the other two possibilities.

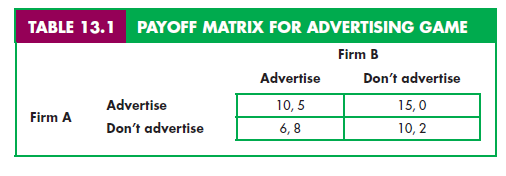
What strategy should each firm choose? First consider Firm A. It should clearly advertise because no matter what firm B does, Firm A does best by advertising. If Firm B advertises, A earns a profit of 10 if it advertises but only 6 if it doesn’t. If B does not advertise, A earns 15 if it advertises but only 10 if it doesn’t. Thus advertising is a dominant strategy for Firm A. The same is true for Firm B: No matter what firm A does, Firm B does best by advertising. Therefore, assuming that both firms are rational, we know that the outcome for this game is that both firms will advertise. This outcome is easy to determine because both firms have dominant strategies.

When every player has a dominant strategy, we call the outcome of the game an equilibrium in dominant strategies. Such games are straightforward to analyze because each player’s optimal strategy can be determined without worrying about the actions of the other players.

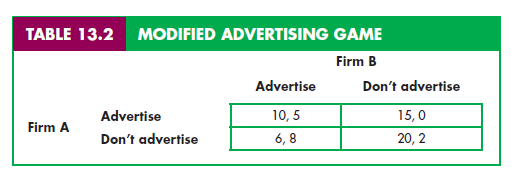
Unfortunately, not every game has a dominant strategy for each player. To see this, let’s change our advertising example slightly. The payoff matrix in Table 13.2 is the same as in Table 13.1 except for the bottom right-hand corner—if neither firm advertises, Firm B will again earn a profit of 2, but Firm A will earn a profit of 20. (Perhaps Firm A’s ads are expensive and largely designed to refute Firm B’s claims, so by not advertising, Firm A can reduce its expenses considerably.)

Now Firm A has no dominant strategy. Its optimal decision depends on what Firm B does. If Firm B advertises, Firm A does best by advertising; but if Firm B does not advertise, Firm A also does best by not advertising. Now suppose both firms must make their decisions at the same time. What should Firm A do?

To answer this, Firm A must put itself in Firm B’s shoes. What decision is best from Firm B’s point of view, and what is Firm B likely to do? The answer is clear: Firm B has a dominant strategy—advertise, no matter what Firm A does. (If Firm A advertises, B earns 5 by advertising and 0 by not advertising; if A doesn’t advertise, B earns 8 if it advertises and 2 if it doesn’t.) Therefore, Firm A can con­clude that Firm B will advertise. This means that Firm A should advertise (and thereby earn 10 instead of 6). The logical outcome of the game is that both firms will advertise because Firm A is doing the best it can given Firm B’s decision; and Firm B is doing the best it can given Firm A’s decision.

Source: Pindyck Robert, Rubinfeld Daniel (2012), Microeconomics, Pearson, 8th edition.