So far we have said nothing about how the market value of an option is determined. We do know what an option is worth when it matures, however. Consider, for instance, our earlier example of an option to buy Amazon stock at $900. If Amazon’s stock price is below $900 on the exercise date, the call will be worthless; if the stock price is above $900, the call will be worth $900 less than the value of the stock. This relationship is depicted by the heavy, lower line in Figure 20.9.
Even before maturity the price of the option can never remain below the heavy, lower- bound line in Figure 20.9. For example, if our option were priced at $20 and the stock were priced at $980, it would pay any investor to sell the stock and then buy it back by purchasing the option and exercising it for an additional $900. That would give an arbitrage opportunity with a profit of $60. The demand for options from investors seeking to exploit this opportunity would quickly force the option price up, at least to the heavy line in the figure. For options that still have some time to run, the heavy line is therefore a lower bound on the market price of the option. Option geeks express the same idea more concisely when they write Lower bound = max(stock price – exercise price, 0).
The diagonal line in Figure 20.9 is the upper bound to the option price. Why? Because the option cannot give a higher ultimate payoff than the stock. If at the option’s expiration the stock price ends up above the exercise price, the option is worth the stock price less the exercise price. If the stock price ends up below the exercise price, the option is worthless, but the stock’s owner still has a valuable security. For example, if the option’s exercise price is $900, then the extra dollar returns realized by stockholders are shown in the following table:
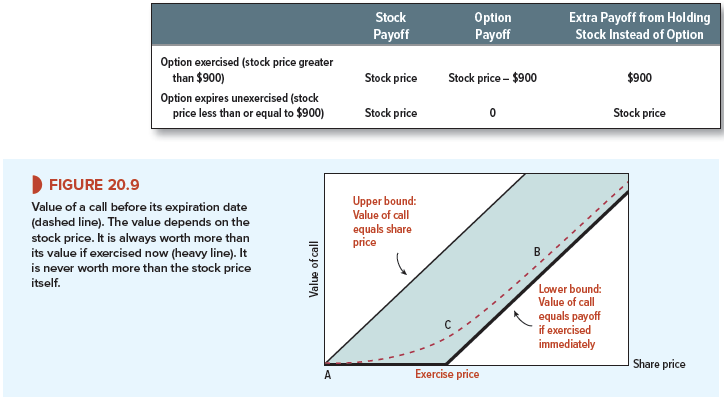
If the stock and the option have the same price, everyone will rush to sell the option and buy the stock. Therefore, the option price must be somewhere in the shaded region of Figure 20.9. In fact, it will lie on a curved, upward-sloping line like the dashed curve shown in the figure. This line begins its travels where the upper and lower bounds meet (at zero). Then it rises, gradually becoming parallel to the upward-sloping part of the lower bound.
But let us look more carefully at the shape and location of the dashed line. Three points, A, B, and C, are marked on the dashed line. As we explain each point you will see why the option price has to behave as the dashed line predicts.
Point A When the stock is worthless, the option is worthless. A stock price of zero means that there is no possibility the stock will ever have any future value.[1] If so, the option is sure to expire unexercised and worthless, and it is worthless today.
That brings us to our first important point about option value:
The value of an option increases as stock price increases, if the exercise price is held constant.
That should be no surprise. Owners of call options clearly hope for the stock price to rise and are happy when it does.
Point B As the stock price increases, the option price approaches the stock price less the present value of the exercise price. Notice that the dashed line representing the option price in Figure 20.9 eventually becomes parallel to the ascending heavy line representing the lower bound on the option price. The reason is as follows: The higher the stock price, the higher is the probability that the option will eventually be exercised. If the stock price is high enough, exercise becomes a virtual certainty; the probability that the stock price will fall below the exercise price before the option expires becomes trivially small.
If you own an option that you know will be exchanged for a share of stock, you effectively own the stock now. The only difference is that you don’t have to pay for the stock (by handing over the exercise price) until later, when formal exercise occurs. In these circumstances, buying the call is equivalent to buying the stock but financing part of the purchase by borrowing. The amount implicitly borrowed is the present value of the exercise price. The value of the call is therefore equal to the stock price less the present value of the exercise price.
This brings us to another important point about options. Investors who acquire stock by way of a call option are buying on credit. They pay the purchase price of the option today, but they do not pay the exercise price until they actually take up the option. The delay in payment is particularly valuable if interest rates are high and the option has a long maturity.
Thus, the value of an option increases with both the rate of interest and the time to maturity.
Point C The option price always exceeds its minimum value (except when stock price is zero). We have seen that the dashed and heavy lines in Figure 20.9 coincide when stock price is zero (point A), but elsewhere the lines diverge; that is, the option price must exceed the minimum value given by the heavy line. The reason for this can be understood by examining point C.
At point C, the stock price exactly equals the exercise price. The option is therefore worthless if exercised today. However, suppose that the option will not expire until three months hence. Of course, we do not know what the stock price will be at the expiration date. There is roughly a 50% chance that it will be higher than the exercise price and a 50% chance that it will be lower. The possible payoffs to the option are therefore
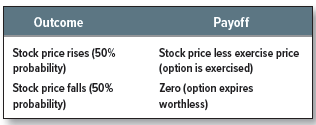
If there is a positive probability of a positive payoff, and if the worst payoff is zero, then the option must be valuable. That means the option price at point C exceeds its lower bound, which at point C is zero. In general, the option prices will exceed their lower-bound values as long as there is time left before expiration.
One of the most important determinants of the height of the dashed curve (i.e., of the difference between actual and lower-bound value) is the likelihood of substantial movements in the stock price. An option on a stock whose price is unlikely to change by more than 1% or 2% is not worth much; an option on a stock whose price may halve or double is very valuable.
As an option holder, you gain from volatility because the payoffs are not symmetric. If the stock price falls below the exercise price, your call option will be worthless, regardless of whether the shortfall is a few cents or many dollars. On the other hand, for every dollar that the stock price rises above the exercise price, your call will be worth an extra dollar. Therefore, the option holder gains from the increased volatility on the upside, but does not lose on the downside.
A simple example may help to illustrate the point. Consider two stocks, X and Y, each of which is priced at $100. The only difference is that the outlook for Y is much less easy to predict. There is a 50% chance that the price of Y will rise to $150 and a similar chance that it will fall to $70. By contrast, there is a 50-50 chance that the price of X will either rise to $130 or fall to $90.
Suppose that you are offered a call option on each of these stocks with an exercise price of $100. The following table compares the possible payoffs from these options:
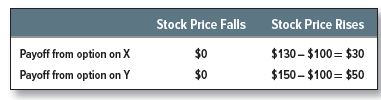
In both cases, there is a 50% chance that the stock price will decline and make the option worthless but, if the stock price rises, the option on Y will give the larger payoff. Because the chance of a zero payoff is the same, the option on Y is worth more than the option on X.
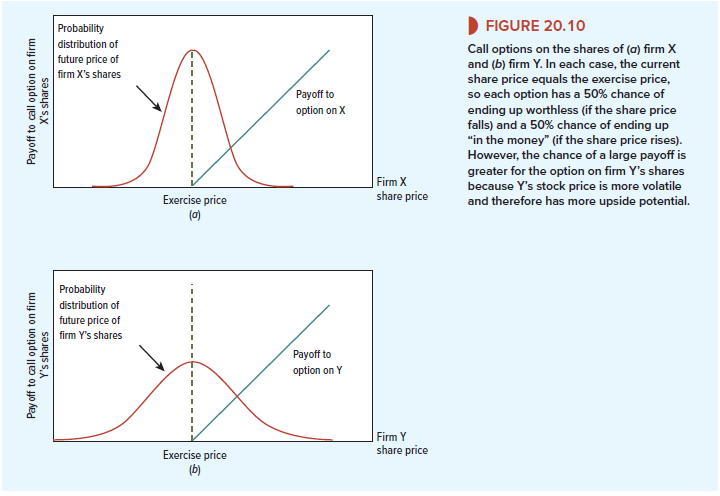
Of course, in practice future stock prices may take on a range of different values. We have recognized this in Figure 20.10, where the uncertain outlook for Y’s stock price shows up in the wider probability distribution of future prices.[2] The greater spread of outcomes for stock Y again provides more upside potential and, therefore, increases the chance of a large payoff on the option.
Figure 20.11 shows how volatility affects the value of an option. The upper curved line depicts the value of the Amazon call option assuming that Amazon’s stock price, like that of stock Y, is highly variable. The lower curved line assumes a lower (and more realistic) degree of volatility.[3]

The probability of large stock price changes during the remaining life of an option depends on two things: (1) the variance (i.e., volatility) of the stock price per period and (2) the number of periods until the option expires. If there are t remaining periods, and the variance per period is a2, the value of the option should depend on cumulative variability a2t.[4]
Other things equal, you would like to hold an option on a volatile stock (high a[5]). Given volatility, you would like to hold an option with a long life ahead of it (large t).
Thus the value of an option increases with both the volatility of the share price and the time to maturity.
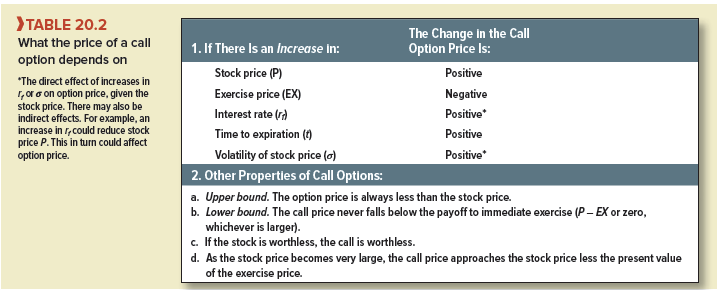
It’s a rare person who can keep all these properties straight at first reading. Therefore, we have summed them up in Table 20.2.
Risk and Option Values
In most financial settings, risk is a bad thing; you have to be paid to bear it. Investors in risky (high-beta) stocks demand higher expected rates of return. High-risk capital investment projects have correspondingly high costs of capital and have to beat higher hurdle rates to achieve positive NPV.
For options it’s the other way around. As we have just seen, options written on volatile assets are worth more than options written on safe assets.[6] If you can understand and remember that one fact about options, you’ve come a long way.
EXAMPLE 20.1 ● Volatility and Executive Stock Options
Suppose you have to choose between two job offers, as CFO of either Establishment Industries or Digital Organics. Establishment Industries’ compensation package includes a grant of the stock options described on the left side of Table 20.3. You demand a similar package from Digital Organics, and they comply. In fact, they match the Establishment Industries options in every respect, as you can see on the right side of Table 20.3. (The two companies’ current stock prices just happen to be the same.) The only difference is that Digital Organics’ stock is 50% more volatile than Establishment Industries’ stock (36% annual standard deviation versus 24% for Establishment Industries).
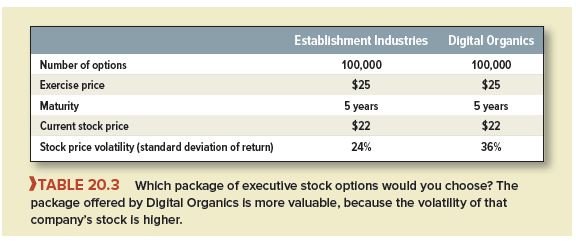
If your job choice hinges on the value of the executive stock options, you should take the Digital Organics offer. The Digital Organics options are written on the more volatile asset and, therefore, are worth more.
We value the two stock-option packages in the next chapter.

You are my aspiration, I possess few blogs and very sporadically run out from to post .
I am constantly thought about this, thankyou for putting up.