We saw in Chapter 12 that in oligopolistic markets, firms often find themselves in a prisoners’ dilemma when making output or pricing decisions. Can firms find a way out of this dilemma, so that oligopolistic coordination and cooperation (whether explicit or implicit) could prevail?
To answer this question, we must recognize that the prisoners’ dilemma, as we have described it so far, is limited: Although some prisoners may have only one opportunity in life to confess or not, most firms set output and price over and over again. In real life, firms play repeated games: Actions are taken and payoffs received over and over again. In repeated games, strategies can become more complex. For example, with each repetition of the prisoners’ dilemma, each firm can develop a reputation about its own behavior and can study the behavior of its competitors.
How does repetition change the likely outcome of the game? Suppose you are Firm 1 in the prisoners’ dilemma illustrated by the payoff matrix in Table 13.8. If you and your competitor both charge a high price, you will both make a higher profit than if you both charged a low price. However, you are afraid to charge a high price because if your competitor charges a low price, you will lose money and, to add insult to injury, your competitor will get rich. But suppose this game is repeated over and over again—for example, you and your competitor simultaneously announce your prices on the first day of every month. Should you then play the game differently, perhaps changing your price over time in response to your competitor’s behavior?
In an interesting study, Robert Axelrod asked game theorists to come up with the best strategy they could think of to play this game in a repeated manner.9 (A possible strategy might be: “I’ll start off with a high price, then lower my price. But then if my competitor lowers his price, I’ll raise mine for a while before lowering it again, etc.”) Then, in a computer simulation, Axelrod played these strategies off against one another to see which worked best.
TIT-FOR-TAT STRATEGY As you would expect, any given strategy would work better against some strategies than it would against others. The objective, however, was to find the strategy that was most robust—that would work best on average against all, or almost all, other strategies. The result was surprising. The strategy that worked best was an extremely simple tit-for-tat strategy: I start out with a high price, which I maintain so long as you continue to “cooperate” and also charge a high price. As soon as you lower your price, however, I follow suit and lower mine. If you later decide to cooperate and raise your price again, I’ll immediately raise my price as well.
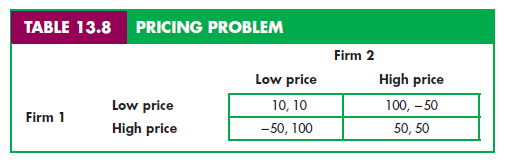
Why does this tit-for-tat strategy work best? In particular, can I expect that using the tit-for-tat strategy will induce my competitor to behave cooperatively (and charge a high price)?
INFINITELY REPEATED GAME Suppose the game is infinitely repeated. In other words, my competitor and I repeatedly set prices month after month, forever. Cooperative behavior (i.e., charging a high price) is then the rational response to a tit-for-tat strategy. (This assumes that my competitor knows, or can figure out, that I am using a tit-for-tat strategy.) To see why, suppose that in one month my competitor sets a low price and undercuts me. In that month he will make a large profit. But my competitor knows that the following month I will set a low price, so that his profit will fall and will remain low as long as we both continue to charge a low price. Because the game is infinitely repeated, the cumulative loss of profits that results must outweigh any short-term gain that accrued during the first month of undercutting. Thus, it is not rational to undercut.
In fact, with an infinitely repeated game, my competitor need not even be sure that I am playing tit-for-tat to make cooperation its own rational strategy. Even if my competitor believes there is only some chance that I am playing tit-for-tat, he will still find it rational to start by charging a high price and maintain it as long as I do. Why? With infinite repetition of the game, the expected gains from cooperation will outweigh those from undercutting. This will be true even if the probability that I am playing tit-for-tat (and so will continue cooperating) is small.
FINITE NUMBER OF REPETITIONS Now suppose the game is repeated a finite number of times—say, N months. (N can be large as long as it is finite.) If my competitor (Firm 2) is rational and believes that I am rational, he will reason as follows: “Because Firm 1 is playing tit-for-tat, I (Firm 2) cannot undercut—that is, until the last month. I should undercut the last month because then I can make a large profit that month, and afterward the game is over, so Firm 1 cannot retaliate. Therefore, I will charge a high price until the last month, and then I will charge a low price.”
However, since I (Firm 1) have also figured this out, I also plan to charge a low price in the last month. Of course, Firm 2 can figure this out as well, and therefore knows that I will charge a low price in the last month. But then what about the next-to-last month? Because there will be no cooperation in the last month, anyway, Firm 2 figures that it should undercut and charge a low price in the next-to-last month. But, of course, I have figured this out too, so I also plan to charge a low price in the next-to-last month. And because the same reasoning applies to each preceding month, the game unravels: The only rational outcome is for both of us to charge a low price every month.
TIT-FOR-TAT IN PRACTICE Since most of us do not expect to live forever, the unravelling argument would seem to make the tit-for-tat strategy of little value, leaving us stuck in the prisoners’ dilemma. In practice, however, tit- for-tat can sometimes work and cooperation can prevail. There are two primary reasons.
First, most managers don’t know how long they will be competing with their rivals, and this also serves to make cooperative behavior a good strategy. If the end point of the repeated game is unknown, the unraveling argument that begins with a clear expectation of undercutting in the last month no longer applies. As with an infinitely repeated game, it will be rational to play tit-for-tat.
Second, my competitor might have some doubt about the extent of my rationality. Suppose my competitor thinks (and he need not be certain) that I am playing tit-for-tat. He also thinks that perhaps I am playing tit- for-tat “blindly,” or with limited rationality, in the sense that I have failed to work out the logical implications of a finite time horizon as discussed above. My competitor thinks, for example, that perhaps I have not figured out that he will undercut me in the last month, so that I should also charge a low price in the last month, and so on. “Perhaps,” thinks my competitor, “Firm 1 will play tit-for-tat blindly, charging a high price as long as I charge a high price.” Then (if the time horizon is long enough), it is rational for my competitor to maintain a high price until the last month (when he will undercut me).
Note that we have stressed the word perhaps. My competitor need not be sure that I am playing tit-for-tat “blindly,” or even that I am playing tit-for-tat at all. Just the possibility can make cooperative behavior a good strategy (until near the end) if the time horizon is long enough. Although my competitor’s conjecture about how I am playing the game might be wrong, cooperative behavior is profitable in expected value terms. With a long time horizon, the sum of current and future profits, weighted by the probability that the conjecture is correct, can exceed the sum of profits from price competition, even if my competitor is the first to undercut. After all, if I am wrong and my competitor charges a low price, I can shift my strategy at the cost of only one period’s profit—a minor cost in light of the substantial profit that I can make if we both choose to set a high price.
Thus, in a repeated game, the prisoners’ dilemma can have a cooperative outcome. In most markets, the game is in fact repeated over a long and uncertain length of time, and managers have doubts about how “perfectly rationally” they and their competitors operate. As a result, in some industries, particularly those in which only a few firms compete over a long period under stable demand and cost conditions, cooperation prevails, even though no contractual arrangements are made. (The water meter industry, discussed below, is an example.) In many other industries, however, there is little or no cooperative behavior.
Sometimes cooperation breaks down or never begins because there are too many firms. More often, failure to cooperate is the result of rapidly shifting demand or cost conditions. Uncertainties about demand or costs make it difficult for the firms to reach an implicit understanding of what cooperation should entail. (Remember that an explicit understanding, arrived at through meetings and discussions, could lead to an antitrust violation.) Suppose, for example, that cost differences or different beliefs about demand lead one firm to conclude that cooperation means charging $50 while a second firm thinks it means $40. If the second firm charges $40, the first firm might view that as a grab for market share and respond in tit-for-tat fashion with a $35 price. A price war could then develop.
Source: Pindyck Robert, Rubinfeld Daniel (2012), Microeconomics, Pearson, 8th edition.

This is a topic which is near to my heart… Thank you! Exactly where are your contact details though?
These are truly enormous ideas in regarding blogging. You have touched some nice things here.
Any way keep up wrinting.
It’s hard to find educated people in this particular topic, but you sound
like you know what you’re talking about! Thanks
I’ve read a few good stuff here. Certainly worth bookmarking for revisiting.
I wonder how a lot attempt you set to create such a excellent informative site.