The Two-Part Tariff

The two-part tariff is related to price discrimination and provides another means of extracting consumer surplus. It requires consumers to pay a fee up front for the right to buy a product. Consumers then pay an additional fee for each unit of the product they wish to consume. The classic example of this strat- egy is an amusement park.12 You pay an admission fee to enter, and you also pay a certain amount for each ride. The owner of the park must decide whether to charge a high entrance fee and a low price for the rides or, alternatively, to admit people for free but charge high prices for the rides.

The two-part tariff has been applied in many settings: tennis and golf clubs (you pay an annual membership fee plus a fee for each use of a court or round of golf); the rental of large mainframe computers (a flat monthly fee plus a fee for each unit of processing time consumed); telephone service (a monthly hook-up fee plus a fee for minutes of usage). The strategy also applies to the sale of prod- ucts like safety razors (you pay for the razor, which lets you consume the blades that fit that brand of razor).

The problem for the firm is how to set the entry fee (which we denote by T) versus the usage fee (which we denote by P). Assuming that the firm has some market power, should it set a high entry fee and low usage fee, or vice versa? To solve this problem, we need to understand the basic principles involved.

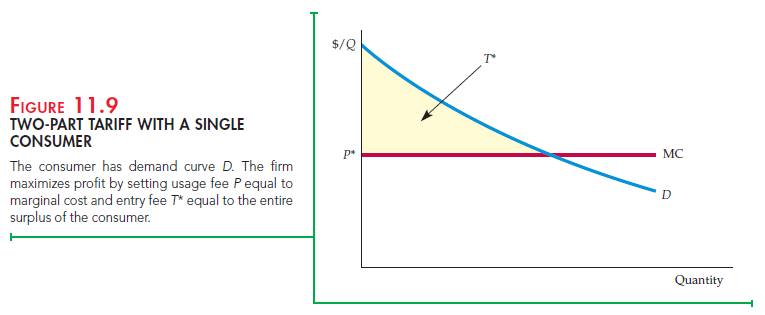
SINGLE CONSUMER Let’s begin with the artificial but simple case illustrated in Figure 11.9. Suppose there is only one consumer in the market (or many con- sumers with identical demand curves). Suppose also that the firm knows this consumer ’s demand curve. Now, remember that the firm wants to capture as much consumer surplus as possible. In this case, the solution is straightforward: Set the usage fee P equal to marginal cost and the entry fee T equal to the total consumer surplus for each consumer. Thus, the consumer pays T* (or a bit less) to use the product, and P* = MC per unit consumed. With the fees set in this way, the firm captures all the consumer surplus as its profit.

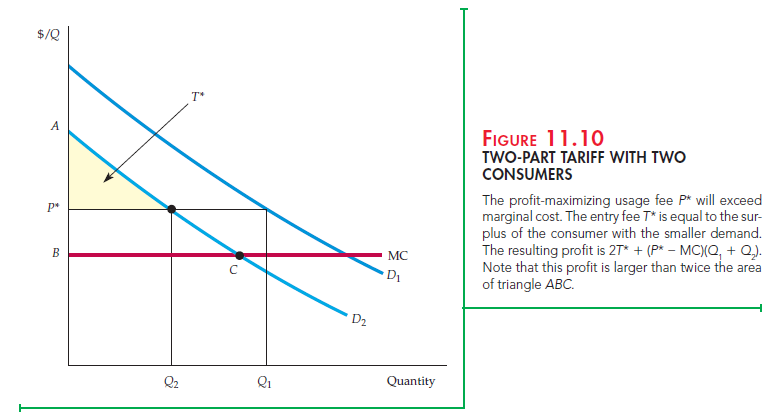
TWO CONSUMERS Now suppose that there are two different consumers (or two groups of identical consumers). The firm, however, can set only one entry fee and one usage fee. It would thus no longer want to set the usage fee equal to marginal cost. If it did, it could make the entry fee no larger than the con- sumer surplus of the consumer with the smaller demand (or else it would lose that consumer), and this would not yield a maximum profit. Instead, the firm should set the usage fee above marginal cost and then set the entry fee equal to the remaining consumer surplus of the consumer with the smaller demand.

Figure 11.10 illustrates this. With the optimal usage fee at P* greater than MC, the firm’s profit is 2T* + (P* − MC)(Q1  + Q2). (There are two consumers, and each pays T*.) You can verify that this profit is more than twice the area of trian- gle ABC, the consumer surplus of the consumer with the smaller demand when P = MC. To determine the exact values of P* and T*, the firm would need to know (in addition to its marginal cost) the demand curves D1  and D2. It would then write down its profit as a function of P and T and choose the two prices that maximize this function. (See Exercise 10 for an example of how to do this.)

MANY CONSUMERS Most firms, however, face a variety of consumers with dif- ferent demands. Unfortunately, there is no simple formula to calculate the opti- mal two-part tariff in this case, and some trial-and-error experiments might be required. But there is always a trade-off: A lower entry fee means more entrants and thus more profit from sales of the item. On the other hand, as the entry fee becomes smaller and the number of entrants larger, the profit derived from the entry fee will fall. The problem, then, is to pick an entry fee that results in the optimum number of entrants—that is, the fee that allows for maximum profit. In principle, we can do this by starting with a price for sales of the item P, finding the optimum entry fee T, and then estimating the resulting profit. The price P is then changed, and the corresponding entry fee calculated, along with the new profit level. By iterating this way, we can approach the optimal two-part tariff.

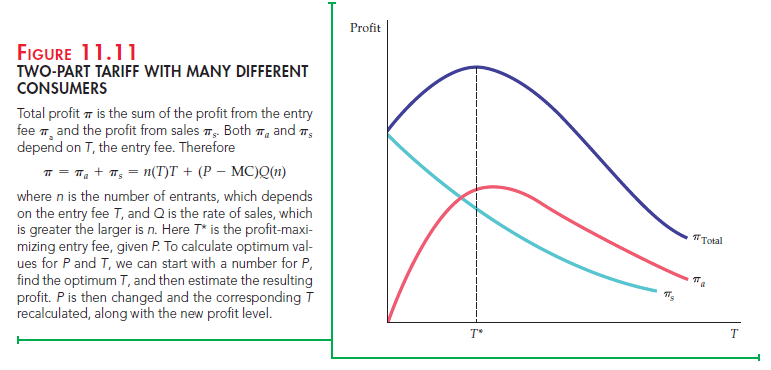
Figure 11.11 illustrates this principle. The firm’s profit p is divided into two components, each of which is plotted as a function of the entry fee T, assuming a fixed sales price P. The first component, p_a, is the profit from the entry fee and is equal to the revenue n(T)T, where n(T) is the number of entrants. (Note that a high T implies a small n. ) Initially, as T is increased from zero, revenue n(T)T rises. Eventually, however, further increases in T will make n so small that n(T)T falls. The second component, p_s, is the profit from sales of the item itself at price P and is equal to (P – MC)Q, where Q is the rate at which entrants purchase the item. The larger the number of entrants n, the larger Q will be. Thus p_s falls when T is increased because a higher T reduces n.

Starting with a number for P, we determine the optimal (profit-maximizing) T*. We then change P, find a new T*, and determine whether profit is now higher or lower. This procedure is repeated until profit has been maximized.

Obviously, more data are needed to design an optimal two-part tariff than to choose a single price. Knowing marginal cost and the aggregate demand curve is not enough. It is impossible (in most cases) to determine the demand curve of every consumer, but one would at least like to know by how much individual demands differ from one another. If consumers’ demands for your product are fairly similar, you would want to charge a price P that is close to marginal cost and make the entry fee T large. This is the ideal situation from the firm’s point of view because most of the consumer surplus could then be captured. On the other hand, if consumers have different demands for your product, you would probably want to set P well above marginal cost and charge a lower entry fee T. In that case, however, the two-part tariff is a less effective means of capturing consumer surplus; setting a single price may do almost as well.

At Disneyland in California and Walt Disney World in Florida, the strategy is to charge a high entry fee and charge nothing for the rides. This policy makes sense because consumers have reasonably similar demands for Disney vaca­tions. Most people visiting the parks plan daily budgets (including expenditures for food and beverages) that, for most consumers, do not differ very much.

Firms are perpetually searching for innovative pricing strategies, and a few have devised and introduced a two-part tariff with a “twist”—the entry fee T entitles the customer to a certain number of free units. For example, if you buy a Gillette razor, several blades are usually included in the package. The monthly lease fee for a mainframe computer usually includes some free usage before usage is charged. This twist lets the firm set a higher entry fee T without los- ing as many small customers. Because these small customers might pay little or nothing for usage under this scheme, the higher entry fee will capture their surplus without driving them out of the market, while also capturing more of the surplus of the large customers.

Source: Pindyck Robert, Rubinfeld Daniel (2012), Microeconomics, Pearson, 8th edition.